Theory Moving coil galvanometer
Let PQRS be a single turn of the coil (Figure: a). A current I flows through the coil. In a radial magnetic field, the plane of the coil is always parallel to the magnetic field. Hence the sides QR and SP are always parallel to the field. So, they do not experience any force. The sides PQ and RS are always perpendicular to the field.
PQ = RS = l, length of the coil and PS = QR = b, breadth of the coil
Force on PQ, F = BI (PQ) = BIl. According to Fleming’s left-hand rule, this force is normal to the plane of the coil and acts outwards.
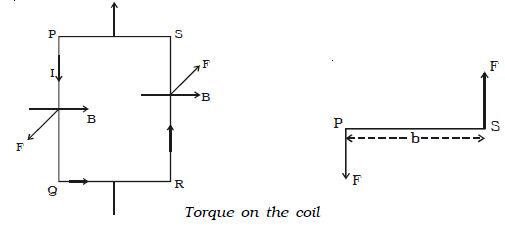
Force on RS, F = BI (RS) = BIl.
This force is normal to the plane of the coil and acts inwards. These two equal, oppositely directed parallel forces having different lines of action constitute a couple and deflect the coil. If there are n turns in the coil, moment of the deflecting couple = n BIl × b (Fig: b) = nBIA
When the coil deflects, the suspension wire is twisted. On account of elasticity, a restoring couple is set up in the wire. This couple is proportional to the twist. If θ is the angular twist, then, moment of the restoring couple = Cθ
where C is the restoring couple per unit twist
At equilibrium, deflecting couple = restoring couple
nBIA = Cθ
I = (C/nBA) x θ
I = K θ where K = C/nBA is the galvanometer constant.
i.e I α θ. Since the deflection is directly proportional to the current flowing through the coil, the scale is linear and is calibrated to give directly the value of the current.