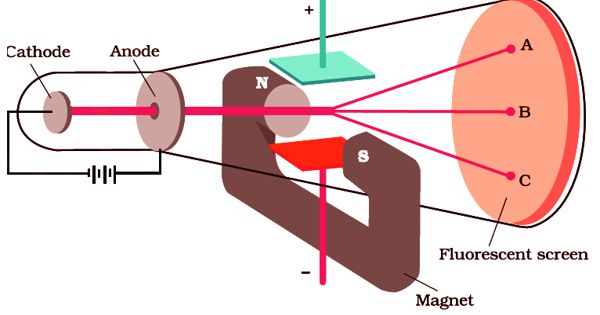
Determination of specific charge (e/m) of an electron – Thomson’s method
In 1887, J.J. Thomson measured the specific charge (e/m) of the cathode ray particles. The specific charge is defined as the charge per unit mass of the particle. Thomson discovered that the value of (e/m) was independent of the gas used and also independent of the nature of the electrodes.
Principle – The fact that the cathode rays (electrons) are deflected by electric and magnetic fields is made use of in this method.
Experimental arrangement
A highly evacuated discharge tube used in this experiment is as shown in Figure. Cathode rays are produced by the discharge between the cathode and the anodes D1 and D2. A thin pencil of cathode-ray comes out through fine pinholes in the anode discs. The cathode rays then pass between two parallel metal plates P1 and P2 and strike the flat face of the tube. This face is coated with suitable fluorescent material. A spot of light is produced at Q But when a potential difference V is applied between P1 and P2, the beam is deflected to point Q1. By the use of a pair of coils, a uniform magnetic field is produced perpendicular to the plane of the paper and outwards throughout the region between P1 P2.
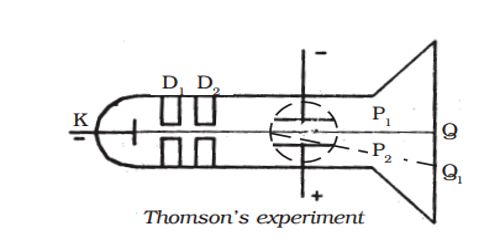
Figure- Thomson’s experiment
Theory
(1) Determination of V
With a given electric intensity between the plates P1 and P2, the magnetic induction B is adjusted until the beam strikes the screen at the original position Q. Then the downward force Ee due to the electric field is balanced by the force Bev due to magnetic induction where ‘e’ is the charge of the cathode ray particle and ‘v’ is the velocity of the cathode rays.
Ee = Bev
v = E/B … … … (1)
(2) Determination of e/m
Now the magnetic induction is switched off. The deflection Q Q1 = y caused by the electric field alone is measured. At the instant when the cathode rays enter the region between the plates P1 and P2, initial velocity in the downward direction u = 0
Acceleration along the downward direction a = Ee/m, where m is the mass of each cathode ray particle.
Time for which the electron moves in the electric field t = l/v; where l is the length of either of the plates and v is the velocity of the electron.
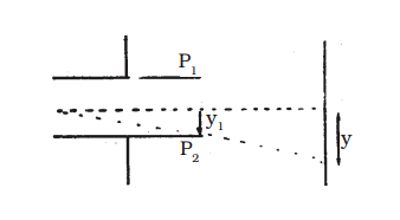
Fig. – Path of an electron in the electric field
The deflection produced on the cathode rays along the downward direction in the electric field is given by,
y1 = ½ (Ee/m) (l/v)2 … …. (2)
Substituting for ‘v’ from equation (1) in equation (2) we get,
y1 = ½ (Ee/m) (l2/E2) B2 = ½ (e/m) (l2B2/e)
The displacement of the spot of light on the screen is given by (Figure 2)
y = K y1, where K is a constant determined by the geometry of the discharge tube. Substituting for y1 we get,
y = K ½ (e/m) (l2B2/E) … …(3)
e/m = 2yE = Kl2B2 …(4)
By substituting the known values in the above relation e/m of an electron can be calculated. The value of e/m calculated using this experiment was found to be 1.7592 × 1011 C kg-1.