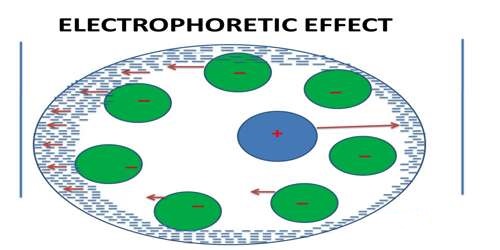
The electrophoretic effect is the effect in which the mobility of ions in solution moving under the influence of an applied electric field is affected by the flow of ions of opposite charge in the opposite direction. The electrophoretic effect is caused by the tendency of the applied electric field to move the ionic atmosphere along with the associated solvent molecules in a direction opposite to the direction in which the central ion (which may also be accompanied by a few solvent molecules) is moving. It may well be imagined that the central ion is moving against the flow of the ionic atmosphere with reduced speed. This effect is called the electrophoretic effect. The ionic electrophoretic effect for solutions of mixed electrolytes of any type is calculated.
Finally, the migration of the ions is opposed by usual frictional resistance of the medium which is again dependent on the dielectric constant of the medium, the radius of the ionic atmosphere and the viscosity of the medium. The attractive forces between the solvent molecules and the ions cause the solvent molecules to be dragged along with the ionic atmosphere. The asymmetry effect is the asymmetrical or irregular distribution of the ion cloud around an ion that occurs from the finite relaxation time when a voltage is applied. It leads to a decrease in the mobility of the ions.
At infinite dilution, the asymmetry and electrophoretic effects are practically zero, and the speed of the ions and hence the molar conductivity is determined by the frictional force of the medium only.
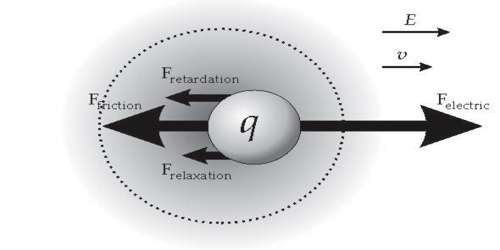
An Ionic atmosphere is a theory that explains the conductivity behavior of solutions (electrolytic solutions), where one charged entity attracts an oppositely charged entity. The electrophoretic effect is the tendency of the applied potential to move the ionic atmosphere which reduces the ionic motion in a solution. The movement of the central ion is slowed down as it moves opposite to the ionic atmosphere, towards the pole.
On the basis of these arguments, Debyc and Huckel were able to derive an expression to explain the variation of conductance with a concentration of the electrolyte. This can be written in the modem form as below-
Λcm = Λ0m – (A + B Λ0m) √C
Λcm = molar conductance of the electrolyte solution of concentration C mol L-1.
Λ0m = molar conductance of the electrolyte solution at infinite dilution.
A and B = constant terms for a particular solvent at a given temperature.