Explain 1 s Electron of the Hydrogen Atom with Integration

The probability P that an electron is a distance r from the nucleus is called the radial distribution function. The hydrogen atom’s is electron radial distribution function is

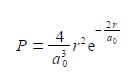
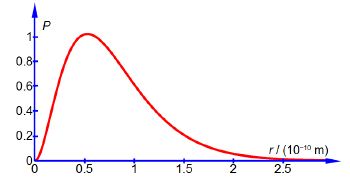
Where a₀ is a constant called the Bohr radius (a₀ = 0.5292 A or a₀ = 0.5292 x 10^-10 m). Below Figure plots the hydrogen atom’s is electron radial distribution function with a₀ = 0.5292 A.

What is the probability of finding the electron at a radius of less than 2a₀, i.e. integrate the above equation from r = 0 to r = 2a₀

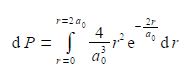
Make use of the standard integral below.

ʃ x² e^bx dx = e^bx [ x²/b – 2x/b² – 2/b³] + C

So,

The required probability comes from integrating the radial distribution function between r = 0 and r = 2a₀. Firstly, take the constant term (4/a₀³) outside the integral.

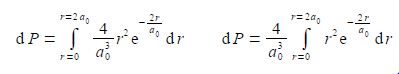
The integral is now recognizable in terms of the standard integral below.

ʃ x² e^bx dx = e^bx [ x²/b – 2x/b² – 2/b³] + C

Using the substitutions x = r and b= —2/a₀ and not forgetting the constant (4/a₀³), the probability integral is

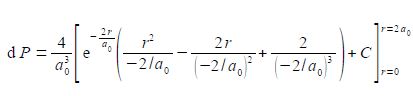
The best way to deal with this complicated looking integral is to clean-up the denominators.

We now substitute in the two limits for our definite integral and the integration constant cancels out.

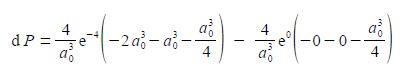
As e⁰ = 1, and cancelling out terms we obtain,

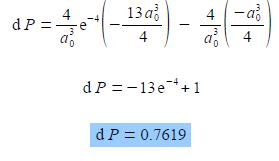
So there is 0.7619 fractional probability (i.e. fraction of unity) that the electron will be within 2a₀ of the nucleus for the is atomic orbital of the hydrogen atom. This is the same as saying there is a 76.19% chance that the 1s electron is between the nucleus and a distance of 2a₀. See Figure for the radial distribution function and this Figure for the 3-dimensional is atomic orbital drawn to enclose 90% of the total probability.