Being startled is something that mathematicians really enjoy. Bonus points if it solves an issue that was previously thought to be unsolvable while also looking good.
You can imagine how happy Alexander Dunn and Maksym Radziwi must have been when they were finally able to upload proof of Patterson’s Conjecture on September 15 of last year. Patterson’s Conjecture is a 45-year-old answer to a problem that dates all the way back to the 19th century.
Dunn told Quanta Magazine that it was “interesting to work on, but really high risk.” I mean, for like four or five months straight, I remember arriving at my office around five in the morning.
What sort of issue would warrant such dedication? Gauss sums it up in two words.
You obviously have some background in number theory if you are familiar with these values, which bear Carl Friedrich Gauss’ name. Gauss was one of the most productive mathematicians in history and was the one who first began experimenting with sums back in the 18th century. Simply said, or as simple as college-level mathematics allows, they are sums of roots of unity: you add all the integers that, for example, cube to equal one. They take the following shape in the quadratic form:
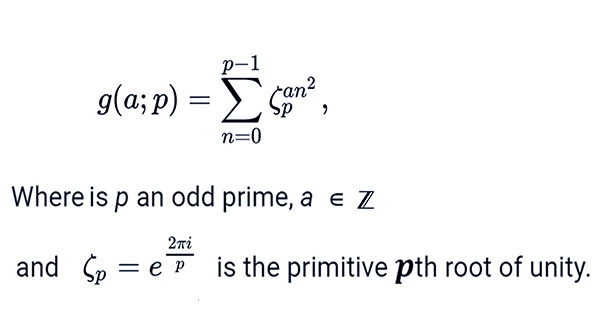
So far, so good, but as you advance a step, from quadratic to cubic, things get really tricky. In one sense, the change is minimal—all we’re doing is swapping out the n2 in the total above for a n3. However, the impact is considerable, which is why it took 175 years for the case to be solved after Ernst Eduard Kummer first started looking into it.
Kummer’s Conjecture: Not that Kummer didn’t make any progress. His 1846 conjecture provided the closest solution to the problem of how the values obtained from cubic Gauss sums are distributed throughout the number line for roughly a century. He hypothesized that for a prime integer p, the outcomes of these cubic Gauss sums may be divided up in a very particular fashion, with half falling between p and 2p, the third falling between p and p, and the last sixth falling between p and 2p.
The conjecture held up when he manually processed cubic Gauss sums corresponding to the first 45 non-trivial prime numbers. However, he was unable to do so; no one could. In fact, mathematicians didn’t consider picking it up again until the early 1950s, with the advent of the computer age.
When they did, they discovered something unanticipated. Kummer had been entirely mistaken.
“Counting the verification described above, the calculation required around 15 million multiplications. P values were added in 200-block increments. In their 1953 publication on Kummer’s Conjecture, John Von Neumann and Herman Goldstine said that the full calculation was performed twice to assure reliability.
The fact that Kummer could be verified would just be a nice bonus. They had picked the problem as a fun method to test a new toy they had been allowed to play with: the first programmable, electronic, general-purpose digital computer, known as ENIAC, developed in 1945. They calculated the findings for primes smaller than a staggering 10,000 with the aid of this digital brain, working with scientist and programmer Hedvig Selberg to somewhat improve on Kummer’s 45 sums.
The pattern also vanished as the number increased. According to Von Neumann and Goldstine, “[The] results would seem to suggest a major deviation from the conjectured densities and a trend towards randomness.”
Patterson’s Conjecture: However, a proof is not always established by a thousand or more cases, and it would be another 15 years before Kummer’s Conjecture was definitively disproven. Samuel Patterson, a number theorist, and Roger Heath-Brown, a graduate student of Patterson’s, were the mathematicians in charge of proving that cubic Gauss sums were really evenly distributed along the number line. Kind of.
Why does that matter? In contrast to his predecessors, Patterson had previously opted to examine what would happen if you added the values of the cubic Gauss sums together. He discovered that a collection of X Gauss sums would total roughly X5/6, which is greater than the square root of X but less than X itself.
That gave him important information. Earlier mathematicians had already demonstrated that a collection of truly random results would add up to roughly X. Finding a total of X5/6 indicated that the sums were essentially random but slightly more likely to be positive than negative due to a little additional element.
If this were the case, it would explain everything, including why Kummer’s results seemed so out of the ordinary and why randomness grew as more primes were calculated. The issue is one of the asymptotes: at smaller scales, the additional component has a discernible impact on the outcomes, but as X grows bigger and bigger, it completely dominates everything else, leaving only randomness.
The only issue was that he was unable to provide any proof. The X5/6 finding replaced Kummer’s Conjecture with Patterson’s Conjecture, but the fundamental question remained unanswered.
However, number theorists are renowned for their singular focus, and Heath-Brown worked on the issue for more than 20 years. He wrote a paper outlining a novel sieve method in 2000, which he thought may be used to ultimately prove Patterson’s Conjecture. Mathematicians use the term “sieve method” to describe algorithms that work through the process of elimination.
He even drew a possible strategy for sharpening and fine-tuning the sieve. Or so he thought, good enough to finally solve the already 150-year-old problem. However, no one was able to achieve it, and we now understand why.
Proof of Patterson’s Conjecture: After very, very difficult work, we were able to demonstrate that 1 Equals 2, Radziwi told Quanta. I was somewhat persuaded that our proof is basically flawed.
However, they were clear that Heath-Brown, like Kummer before him, had been disproven. His “enhanced” sieve was nothing of the such, and Radziwi and Dunn would have to return to the original cubic big sieve in order to answer Patterson’s conjecture.
According to Radziwi, “I believe it was the primary reason why nobody [solved Patterson’s Hypothesis], since this [Heath-Brown] conjecture was deceiving everyone.” “I believe that Heath-Brown would likely figure out how to do it if I told him that his conjecture is incorrect.”
Knowing where earlier attempts had fallen short, Radziwi and Dunn succeeded where others had failed, and their paper finally puts to rest a problem that has plagued number of theorists for the better part of two centuries. And there is only one tiny issue remaining to resolve before it is absolutely, positively proved beyond a reasonable doubt.
The pair write in their work that “a dispersion estimate for cubic Gauss sums is a key part in our proof.” Our finding is conditional because of this estimate, which is based on the Generalized Riemann Hypothesis.
Now that the Riemann Hypothesis has been proven, everything is established. Simple as pie, right?
















