What is Momentum in Kinetic Energy Theorem?

When a ball bounces off a wall, it is reflected with the same speed but travels in the opposite direction: v → -v The kinetic energy is constant, and the net work is done on the ball by the wall is zero. Since Δv = v_f – v_i = -2v = a_ave Δt, we define the impulse as force * time,

J = F Δt ….. ……….. ……(1)

So the momentum of an object with mass in as mass * velocity is

p = mv ……. …….. ……(2)

Newton’s second law, F = ma , can then be written,

J = Δp ……. …….. ……(3)

This relationship is somewhat analogous to the work-kinetic energy theorem. It allows us to describe changes in the velocity of an object without knowing details of the forces [the magnitudes and direction and for how long they last]. For the ball bouncing off the wall, we do not know the magnitude of the force exerted by the wall on the ball or the duration of the force; however, the impulse of the force has a known value, J = – 2mv. The unit of momentum and impulse are [J] = [p] = N • s = kg • m/s.

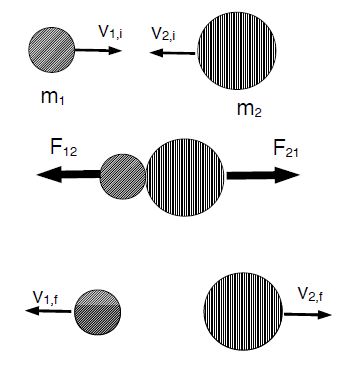
We have two objects [pucks] sliding on (idealized) ice and traveling at velocities v_1,i and v_2,i respectively. They collide and exert forces F₁₂ and F₂₁ on each other. These two forces are an action-reaction pair so that F₁₂ = – F₂₁. After the collision, they travel at velocities v_1f and v_2f. Note that there no external forces, for example the gravitational force in the case when the surface is inclined.

We have for the impulses,

F₁₂ Δt = m Δv₁ = m₁ (v_1,f — v_1,i)

F₂₁ Δt = m Δv₂ = m₂ (v_2,f — v_2,i)

Newton’s third law states F₁₂ = -F₂₁, or F₁₂ + F₂₁ = 0, so that the addition of the two equations yields

(F₁₂ + F₂₁) Δt = m₁ (v_1,f — v_1,i) + m₂ (v_2,f — v_2,i) = 0 or,

m₁ v_1,i + m₂ v_2,i = m₁ v_1,f + m₂ v_2,f

We define the total momentum of the system [mass m₁ and mass m₂],

p_tot = m₁ v₁ + m₂ v₂

We thus see that the total momentum is the same before and after the collision. We say that the total momentum is conserved. No detailed knowledge of the forces is necessary.