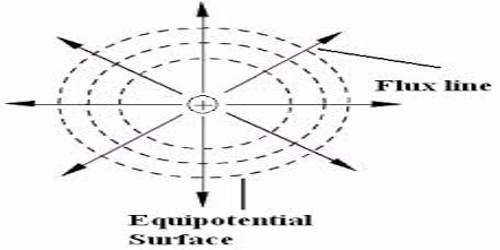
Equipotential surface
We have come to know that potential everywhere on earth’s surface is same (zero), as the surface of the earth is a good electric conductor. On the surface of the conductor potential difference cannot exist, because if there is potential gradient an electric field will act on the surface and electrons of the surface will distribute in such way, due to the influence of the electric field, that electric field disappears. The total charge of the conductor whether positive or negative or the conductor is chargeless or whatever may be the actual potential of the conductor with respect to an object, potential on the surface all cases will be equal everywhere.
So, it can be said that the surface or volume becomes such that its potential is everywhere same, then that surface or volume is called equipotential surface or volume.
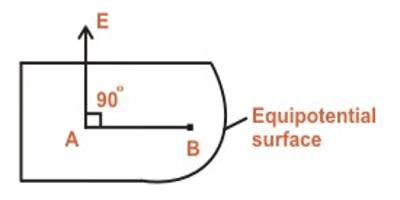
For example, consider two points A and B on an equipotential surface as shown in the figure.
VB – VA = 0
VB = VA
It may be noted that an equipotential surface may be the surface of a material body or a surface drawn in an electric field.