Describe Reflectance and Transmittance

The reflectance and transmittance are defined in terms of the rate of energy flow incident on unit area of the interface, S_i • (- e_i) = S_i cos θ_i. Thus the reflectance and transmittance are

R = (S_r cos θ_r)/ (S_i cos θ_i);     T = (S_t cos θ_t)/ (S_i cos θ_i)

Where,

S = ε E² v_p ≈ (n² ε₀) E² (c/n)

is the magnitude of the Poynting vector, and the cos θ factors take account of the projected area of the interface as seen by the incoming and outgoing waves. Hence

R = |r|²;      T = (n_t/n_i) (cos θ_t / cos θ_i) t² = 1 – R

For the case of internal reflection we see that as θ_i → θ_c both amplitude reflection coefficients obey r → 1, while the amplitude transmission coefficients remain finite. However, because cos θ_t → 0 as θ_i → θ_c, while cos θ_i → cos θ_c which is finite, from Equation we must have T → 0 as θ_i → θ_c. It is straightforward to show that R + T = 1, as required by energy conservation. The reflectance is shown in below Figure for the cases of (a) external reflection and (b) internal reflection.

Notice that for the case of external reflection shown in Fig (a) and grazing incidence, i.e.. θ_i → 90°, the reflectance approaches 100% (R → 1). This phenomenon is used in the design of imaging X-ray space telescopes, and a typical design based on XMM-Newton is sketched in below Figure.

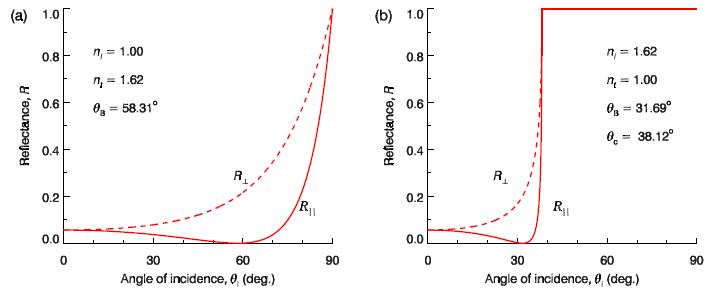
Figure: Reflectance for (a) external reflection off flint glass and (b) internal reflection within flint glass.