The electro motive force (abbreviation emf, symbol Ɛ, unit V) is what drives a current around a circuit. Examples of sources of emf include batteries, piezoelectric crystals, solar cells and electrical generators (alternators and dynamos). In electrical generators an engine fuelled, for example by coal, oil or nuclear fission, or a water turbine in a hydroelectric plant or a wind turbine in a wind farm, moves conductors through a magnetic field to produce electricity.
The purpose of a source of emf is to maintain a potential difference across its terminals. Within a source of emf there is a non-conservative “electro-motive” force FEM acting on positive charges and pushing them towards the positive terminal “A”, and on negative charges pushing them towards the negative terminal “B” (see Fig). Integrating the electro-motive force per unit charge EEM = FEM/q from the negative terminal through the source of emf to the positive terminal gives the emf,
Ɛ = ʃinside source EEM. Dr
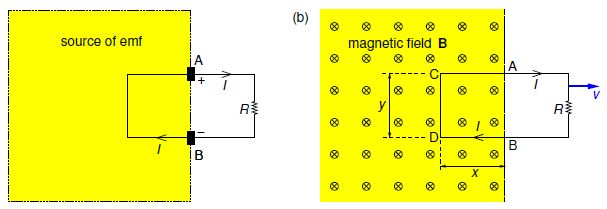
Figure: (a) Source of emf: inside the source the electro-motive “force” pushes positive charge towards the positive terminal; outside the source the electro-motive “force” is zero and the electrostatic force pushes positive charge towards the negative terminal. (b) Motional emf: the circuit is pulled to the right at constant velocity v through a uniform magnetic field B (pointing into the page) which causes current I to flow.
The electro-motive force exists only inside the source of emf. Within the source it is in the opposite direction to the (conservative) electrostatic force qEES which pushes positive charges away from the positive terminal, and the electro-motive and the electrostatic forces cancel each other out. Thus, if we integrate the vector sum of the two forces per unit charge, Etotal = EEM + EES around a dosed loop from the negative terminal through the source of emf to the positive terminal then outside the source back to the negative terminal we also get the emf because EEM = 0 outside the source and ʃ EES • dr = 0, and so
Ɛ = ʃvia source Etotal . dr
From now I shall represent Etotal simply by E and remember it is in general the vector sum of the electrostatic field and the non-conservative electric field associated with a source of emf.











