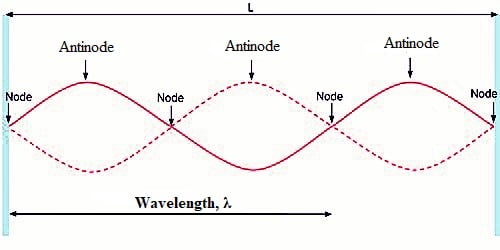
When two, identical, progressive waves of equal amplitudes and equal wavelengths and traveling in the similar medium, along the similar straight line, but in opposite directions, interfere, and then the wave formed is called a standing wave or a stationary wave.
Some Characteristics of stationary waves:
(a) The waveform remains stationary. They are formed due to the intervention of two identical progressive waves traveling along a similar path but in contradictory directions. Compressions and rarefactions do not travel forward as in progressive waves. They appear and disappear alternately, at a similar place.
(b) Nodes and antinodes are formed alternately. There are some particles of the medium which do not at all vibrate, i.e. their amplitude is zero. These are called nodes; some particles vibrate with utmost amplitude and are called antinodes. The amplitude of vibration increases steadily from zero to utmost from a node to an antinode. The points where displacement is zero are called nodes and the points where the displacement is maximum are called antinodes.
(c) Pressure changes are maximum at nodes and minimum at antinodes. All the particles except those at the nodes, execute simple harmonic motions of the same period. The amplitude of each particle is not the same, it is maximum at antinodes decreases gradually and is zero at the nodes. The velocity of the particles at the nodes is zero. It increases gradually and is maximum at the antinodes.
(d) The distance between any two consecutive nodes or antinodes is A equal to λ/2, whereas the distance between a node and its adjacent antinode is equal to λ/4. They move neither forward nor backward. All the particles of the medium except those at the nodes vibrate simply harmonically with a time period equivalent to that of the component waves. There is no onward motion of the disturbance from one particle to the adjoining particle and so on beyond this particular region.
(e) There is no transfer of energy. All the particles of the medium pass through their mean position simultaneously twice during each vibration. In a stationary wave, the energy is not transported from one point to another. There is no net transport of energy in the medium. The total energy connected with a stationary wave is twice the energy of each of the incident and the reflected wave. There is no flow of energy along the stationary waves.
(f) Particles in the same segment vibrate in the same phase and between the neighboring segments, the particles vibrate in opposite phase. There is no progressive alter of the segment from element to element. In fact, all the particles in any one segment or loop vibrate in the similar phase, while particles in any two adjacent segments are in contradictory phase.
(g) All the particles in a similar loop have a similar phase at a given instant. In a given segment, the particles accomplish their maximum or minimum velocity and acceleration at the same instant. The disturbance is confined to an exacting region between the starting point and the reflecting point of the wave.
(h) Stationary waves can be produced by the interference of either longitudinal or transverse waves. The amplitude varies gradually from zero at the nodes to the maximum at the antinodes. The distance between two successive nodes or antinodes is equal to half the wavelength.
(i) In a stationary wave, the medium splits up into a number of segments. Each segment is vibrating up and down as a whole. All the particles in one exacting segment vibrate in the same phase. The conditions of crest and trough simply appear and disappear in a fixed location to be followed by the contradictory situation after every half time period.