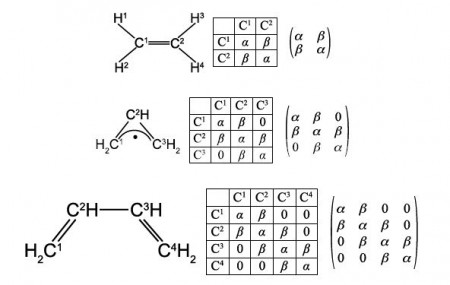
Matrices can be used to represent molecular Chemical Connectivity to show, using Maths, those atoms which are bonded to one another. For example, ethene, or ethylene, with the atoms numbered for easy reference, is shown in below Figure.

A connectivity table is shown below, Figure, as follows: on the diagonal elements of the matrix, place an α (Greek alpha); on the off-diagonal elements where an atom is bonded to the indicated atom place a p β (Greek beta); and on the off-diagonal elements where an atom is not bonded to the indicated atom place a 0 (zero). The Chemical Connectivity table can be conveniently written in matrix form and we will use this type of matrix later on for carrying out some symmetry operations and also we will use some related matrices for molecular orbital calculations.

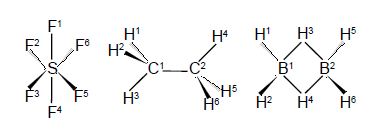
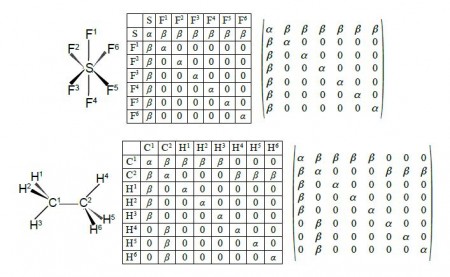
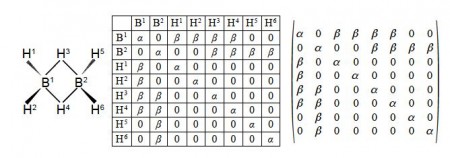
Firstly, draw up similar connectivity matrices for the following molecules: sulfurhexafluoride (SF6), ethane, and diborane (B2H2), all shown in below Figure.
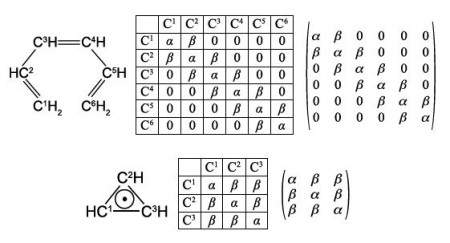
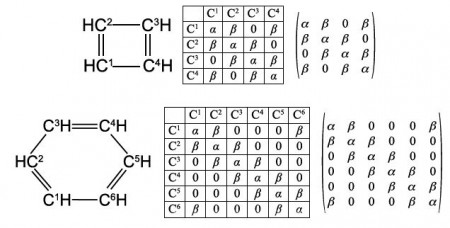
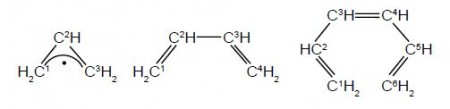
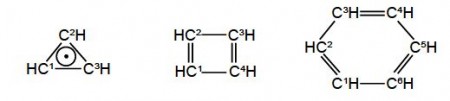
Secondly, draw up connectivity matrices for the following unsaturated molecules and radicals; ethene and those shown in below Figures but now only consider the heavy atoms and ignore the Chemical Connectivity of the hydrogen atoms.
Solution
Firstly we are asked to draw up Chemical Connectivity matrices for SF6, ethane and diborane. We note that although ethane and diborane have the similar empirical formulae their connectivities are very different.
Secondly, we are asked to draw up Chemical Connectivity matrices for the unsaturated acyclic molecules and radicals ethene, allyl radical, butadiene, and hexatriene; and then their cyclic counterparts cyclopropenyl radical, cyclobutadiene and benzene. For all these species we will consider only the carbon atoms.