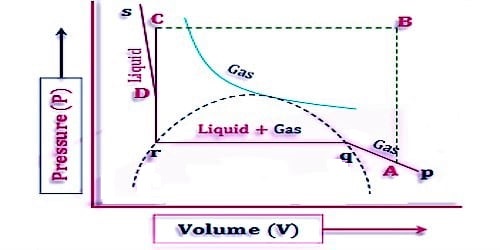
At the critical temperature, the boundary between the liquid phase and gas phase disappears and the densities of the substance in the two phases are the same. These observations led Andrews to believe that there is no difference between the liquid and the gas at the critical temperature. Continuity of State is the property of a transition between two states of matter, as between gas and liquid, during which there are no abrupt changes in physical properties.
He went on further to state that the ordinary gaseous and liquid states are only widely separated states of matter and that one state may be made to pass to the other by a course of continuous physical changes representing nowhere any break of the continuity. In other words, the transformation from the gaseous state to the liquid state or vice versa may be made gradually, without pawns through the phenomenon of condensation, i.e., nowhere during the transformation two phases will co-exist. This is Andrews theory of the “Continuity of State”
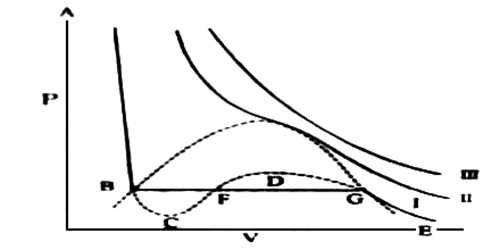
Figure 1
The idea of the continuity of state may be understood more clearly with reference to Figure 1, where the parts BC and GD of curve I (and of similar curves below the critical temperature) can be realized experimentally. If a gas is compressed isothermally in a perfectly clean and smooth vessel the volume decreases along the isothermal EG until the point G is reached, when liquefaction should start. However, it may so happen that liquid does not form and the further increase in pressure produces a decrease in volume along the line GD. Along GD the gas may be termed as supersaturated or supercooled vapor. Any attempt at the increase of pressure beyond D results in sudden liquefaction. Similarly, section BC of the isotherm may be realized; i.e. with the reduction of pressure the volume of the liquid increases along BC without vaporization starting at B. The system is unstable along BC in the sense that a slight disturbance would be sufficient to cause the system to revert spontaneously to the stable state, with the liquid and vapor in equilibrium. The part CFD cannot be realized experimentally as this represents the impossible situation that the volume increases when the pressure is increased.
In spite of these them is an element of continuity from the gaseous state to the liquid state as the continuous curve ABCFDGE represents the P-V relation of the substance in both the states. We know that the van der Waals equation mathematically represents curve I, whereas it is impossible to get an analytical expression which will correspond to the isothermals below the critical temperature as obtained by Andrews.
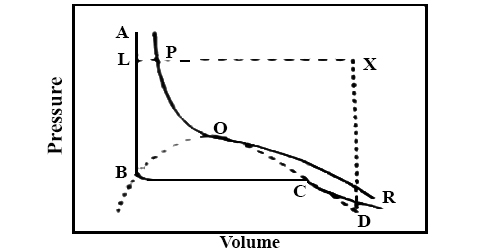
Figure 2
The essential feature of the idea of the continuity of state may be demonstrated from yet another point of a view. Consider Andrews isothermal for a gas, Figure 2. If the gas is heated, keeping the volume constant, the pressure will rise along DX. Now decrease the temperature keeping the pressure constant the volume will decrease along XL. The point L is on that portion of the isothermal ABCD, which represents the liquid state. Thus the substance which was gaseous at D has been converted into the liquid at L, but at no time there was more than one phase.
Somewhere along the line XL, most probably at P, the gas has turned into liquid; to the left of the critical isotherm PQR it is all liquid but to the right it is gaseous. The change from the gaseous to the liquid state has been gradual and transition may be regarded as continuous.
There is no sharp transition between liquid and gaseous states in contrast to that observed on melting a solid. In other words, gas and liquid states for chemical matter form a continuity of states.
The law of corresponding states introduces a substantial proposition in the treatment of very nonideal gases. The uniform actions of such gases in terms of the reduced variables should not ambiguous the fact that critical data, which are characteristic of each gas, are inherent in the reduced variables.