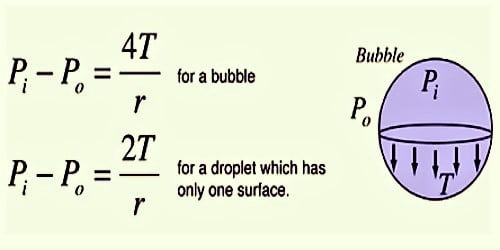
A soap bubble has two liquid surfaces in contact with air, one inside the bubble and the other outside the bubble. In balance, the air pressure surrounded by a soap bubble is enhanced than the atmospheric pressure. Consider a soap bubble of radius R and surface tension T. This surplus pressure is formed due to the surface tension of the soap solution. There are two free surfaces of the soap bubble. Due to surface tension, the molecules on the outside layer experience the net force in the internal way usual to the surface.
If R is the radius of the bubble and T is its surface tension, then for the uncharged bubble in stability Force due to excess pressure = force produced due to surface tension. Therefore, there is more pressure inside than outside.
Therefore the force due to surface tension = 2 x 2πrT
At equilibrium, P πr2 = 2 x 2πrT
So, P = 4T/r
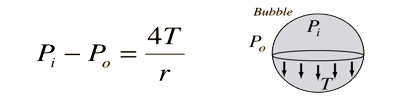
Thus the excess of pressure inside a drop is inversely proportional to its radius, P ∞ 1/r. As P ∞ 1/r, the pressure needed to form a very small bubble is high. The reality that air has to be blown into a drop of soap solution to make a bubble should commend that the pressure within the bubble is better than that exterior. This explains why one needs to blow hard to start a balloon growing. Once the balloon has grown, less air pressure is needed to make it expand more. This is, in reality, the case: this surplus pressure creates a force that is just impartial by the inmost pull of the soap layer of the bubble due to its surface tension.