Experiment: Torque experienced by a Current Loop in Uniform Magnetic Field

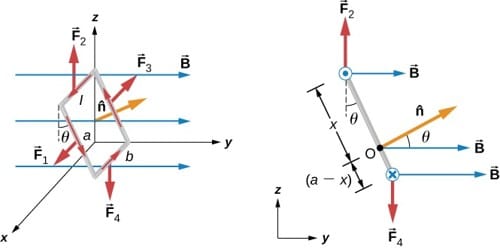
A uniform magnetic field exerts no net force on a current loop but it does exert a net torque. Torque (τ) is the twisting force that tends to cause rotation. The axis of rotation is the point where the object rotates.

Torque experienced by a current loop in a uniform magnetic field

Let consider a rectangular loop PQRS of length l and breadth b (Figure). It carries a current of I along PQRS. The loop is placed in a uniform magnetic field of induction B. Let θ be the angle between the normal to the plane of the loop and the direction of the magnetic field.

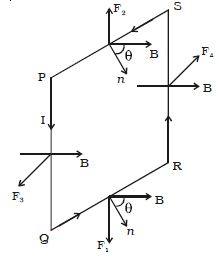
Fig: Torque on a current loop placed in a magnetic field

Force on the arm QR, ^→F₁ = ^→I(QR) × ^→B

Since the angle between ^→I(QR) and ^→B is (90⁰ – θ),

The magnitude of the force F₁ = BIb sin (90⁰ – θ)

F₁ = BIb cos θ

Force on the arm SP, ^→F₂ = ^→I(SP) × ^→B

Since the angle between ^→I(SP) and ^→B is (90⁰ + θ),

The magnitude of the force F₂ = BIb cos θ

The forces F₁ and F₂ are equal in magnitude, opposite in direction and have the same line of action. Hence their resultant effect on the loop is zero.

Force on the arm PQ, ^→F₃ = ^→I(PQ) × ^→B

Since the angle between ^→I(PQ) and ^→B is 90⁰,

The magnitude of the force F₃ = BIl sin 90⁰ = BIl

F₃ acts perpendicular to the plane of the paper and outwards.

Force on the arm RS, ^→F₄ = ^→I(RS) × ^→B

Since the angle between ^→I(RS) and ^→B is 90⁰,

The magnitude of the force F₄ = BIl sin 90⁰ = BIl

F₄ acts perpendicular to the plane of the paper and inwards.

The forces F₃ and F₄ are equal in magnitude, opposite in direction and have different lines of action. So, they constitute a couple.

Hence, Torque = BIl × PN = BIl × PS × sin θ (Figure)

= BIl × b sin θ = BIA sin θ

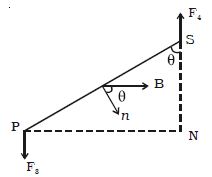
Fig: Torque

If the coil contains n turns, τ = nBIA sin θ

So, the torque is maximum when the coil is parallel to the magnetic field and zero when the coil is perpendicular to the magnetic field.

Torque, τ = F × r

Where, F = force applied and, r = distance between the center of the axis of rotation and to the point where force is applied.

Mathematical Explanation with Example:

Motors are the most common function of the magnetic force on current-carrying wires. Motors contain loops of wire in a magnetic field. When current is passed through the loops, the magnetic field exerts torque on the loops, which rotates a shaft. Electrical energy is converted into mechanical work in the procedure.

[Find the maximum torque on a 100-turn square loop of a wire of 10.0 cm on a side that carries 15.0 A of current in a 2.00-T field.]

Strategy – Torque on the loop can be found using τ = NIABsinθτ = NIAB sin⁡θ. Maximum torque occurs when θ = 90º and sin θ = 1.

Solution

For sin θ = 1, the maximum torque is

τmax=NIABτmax=NIAB

Entering known values yields

τmax = (100)(15.0 A)(0.100 m2)(2.00 T) = 30.0 N⋅mτmax=(100)(15.0 A)(0.100 m2)(2.00 T) = 30.0 N⋅m.