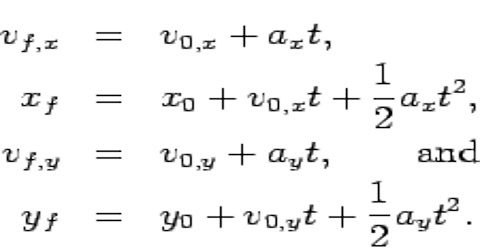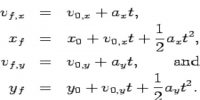First Equation of Dimensional Motion

First Equation of Dimensional Motion

In one dimensional motion a body moves along a straight line. So quantities associated with motion, for example displacement, velocity, acceleration etc., have only one component (moving along X-axis will have X-component and Y and Z components will be zero). In deriving equations of linear motion we will consider that the body is moving along X-axis. In that case subscripts associated with different quantifies of motion may be omitted. Normally, v_x will be represented by v and a_x by a.

(i) Equation of position or displacement of a body moving with uniform velocity.

(s = vt) or x = x₀ + v_xt.

Let an object move with uniform velocity v. The object travels in 1 sec a distance, v x 1. So, in t seconds, it travels the distance, vt.

Let the distance travelled in t sec be, s.

So, S = vt … … … (1)

For one dimensional motion, say along X-axis, if x₀ is the position at t = 0 and final position at t = t is x. then s = x – x₀.

From equation (1), we get

x – x₀ = v_xt, here v_x is the uniform velocity along X-axis.

or, x = x₀ + v_xt … … … (ii)

Similarly, for Y and Z-axis, we get,

y = y₀ + v_yt and z = z₀ + z_yt

(ii) Relation between displacement, velocity and time of a body moving with variable velocity.

If the body is in motion with variable or non-uniform velocity, then average velocity is taken.

Let at time t = 0, the initial position of the body be x₀ and at time t its position be x.

So, displacement of the body, ∆x = x – x₀ and elapsed time, ∆t = t – 0 = t.

We know,

average velocity, v = ∆x/∆t = (x – x₀)/∆t

or, (x – x₀) = vt

or, x = x₀ + vt … … … (2)

If the body start from origin, x₀ = 0, then equation (2) becomes: x = vt

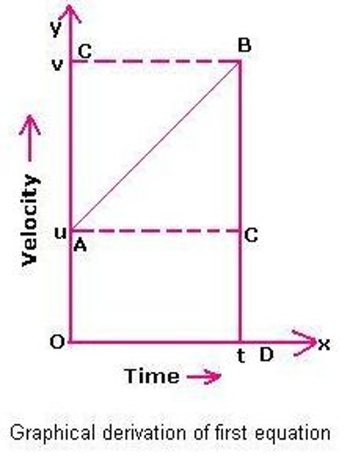
(iii) Relation between displacement, velocity and time of a body moving with uniform acceleration.

Let an object move with uniform acceleration along X-axis. If at time interval ∆t the displacement of the object is ∆x, then average velocity,

v = ∆x/∆t = (x – x₀)/t .. … …. (3)

Here at t = 0; at the start, the position of the objects is x₀ and at time t, the position is x.

Now, average velocity of an object moving with uniform acceleration is halt of the sum of the initial and final velocities i.e.,

v = (v₀ + v)/2; here v₀ = initial velocity and v = final velocity.

Putting this value in equation (3), we get;

(v₀ + v)/2 = (x – x₀)/t

(x – x₀) = ½ (v₀ + v)t

or, x = x₀ + ½ (v₀ + v)t … … … (4)

If displacement ∆x = x – x₀ = α; then equation (4) can be written as:

S = ½ (v₀ + v)t