Predicting Precipitation
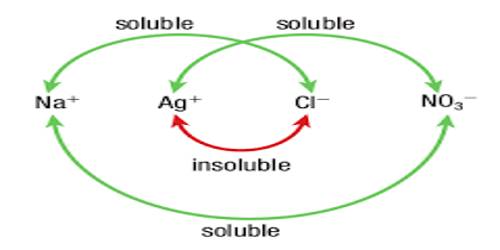
It is known that when a solution of silver nitrate is added to a solution of sodium chloride or any other chloride a precipitate of silver chloride is formed. In fact, this is a test for the presence of chloride ion in solution. Similarly, the presence of sulphate ion in a solution is indicated by the formation of the precipitate on addition of barium chloride solution to the test solution. It has been found, however, that if the solutions are extremely dilute precipitation may not occur. The solubility product value of the sparingly salt which forms the precipitate may be used to predict whether precipitation will occur or not.
Suppose, for example, we want to find out if a precipitate will form when a solution of lead nitrate of a certain concentration will form a precipitate of lead sulphate when a solution of sodium sulphate is added to it. First of all, we have to consider the equilibrium
PbSO4 (s) + aq = Pb2+ (aq) + SO42- (aq)
The solubility product of lead sulphate is given by,
Ksp = [Pb2+] [SO42-] = 1.7 x 10-8 at 250 C.
In a solution of lead sulphate as long as the quantity on the right-hand side of the solubility product expression, i.e., [Pb2+] [SO42-] is less than 1.7 x 10-8 there will be no precipitation. If [Pb2+] [SO42-] is equal to greater than Ksp = 1.7 x 10-8 precipitation occurs.
For any sparingly soluble salt the expression written for Ksp, is also known as the reaction quotient or ion product. For example, for Mg(OH)2 the reaction quotient is [Mg2+] [OH–]2 and for Ca3(PO4)2 the reaction quotient is [Ca2+]3 [PO43-]2. If the reaction quotient, which is designated as Qc, is less than Ksp, there will be no precipitation. If, however, Qc, is equal to or greater that Ksp precipitation occurs.