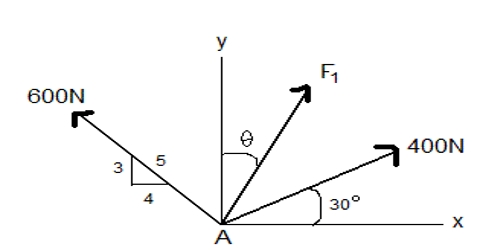
Determination of Magnitude of Resultant

Determination of magnitude of the resultant:

Suppose the magnitude of the resultant is R and AOC = α is an acute angle (Figure). Now from point B let us draw normal BN on the extended line of OA which intersects the line OA at N.

Now, AB and OC are parallel

so, AOC = BAN = α

Again in, ∆OBN, ONB = 90⁰

so, OB² = ON² + BN² = (OA + AN)² + BN²

= OA² + 2OA.AN + AN² + BN²

But, AB² = AN² + BN² [here, ∆BNA is a right angle triangle]

or, OC² = AN² + BN² [AB = OC]

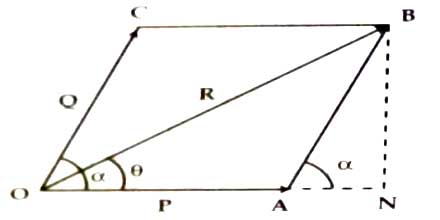
Now, from trigonometry,

AN = AB cos α = OC cos α

so, OB² = OA² + OC² + 2OA.AB cos α

or, OB² = OA² + OC² + 2OA.OC cos α

or, R² = P² + Q² + 2PQ cos α

then, R = √(P² + Q² + 2PQ cos α) … … … (i)

Direction of the Resultant:

Suppose the resultant R makes an angle θ with P; i.e., AOB = θ

so, in the right angle ∆OBN,

tan θ = BN/ON = BN/(OA+AN)

= AB sinα / (OA+AB Cosα) (here, sinα = BN/AB and cosα = AN/AB)

so, tanθ = Q sinα / (P+Qcosθ) … … … (ii)

Hence, from equation (i) and (ii), we get R and θ recpectively;

Vectors AB and BC represent 4 km and 10 km displacements respectively along the North and the East. According to the law of triangle the resultant of the displacement vector AC is drawn.