Explain Superficial Expansion and Coefficient of Superficial Expansion

The area of a solid is increased with an increase of temperature. It is called superficial expansion. Whenever there is an increase in the size of a body due to heating, then the body is said to be expanded and the fact is known as expansion.

Let the initial surface area of a solid at θ₁ temperature = A₁

When the temperature is increased to θ₂ the final surface area =A₂

So, Increase in temperature = θ₂ – θ₁

And the increase in area = A₂ – A₁

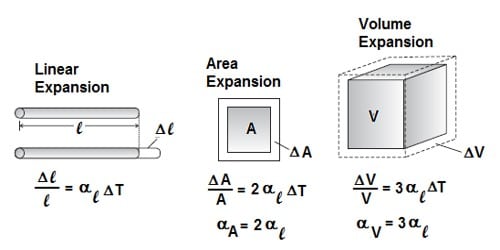
The coefficient of superficial expansion is expressed by the symbol β (beta). The amount by which unit area of a material increases when the temperature is raised by one degree is called the coefficient of superficial (i.e. area) expansion and is represented by β (Greek beta).

Superficial expansion, β = [(A₂ – A₁) / A₁(θ₂ – θ₁)] ….. ………. ………(1)

[Increase in surface area / (Initial area x increase of temperature)]

In equation (1) if the surface area, A₁ = 1 m² and the increase of temperature (θ₂ – θ₁) = 1K is considered, then the increase in surface area, β (beta) = A₂ – A₁= increase in surface area. If the temperature increases, then the volume of the substance also increases. Usually, this is known as thermal expansion.

Superficial Expansion of Solids: The coefficient of superficial expansion is defined as the ratio of increase in area to its original area for every degree increase in temperature.

Coefficient of superficial expansion = Increase in area / (Initial area – Rise in temp) (°C) = 2 × coefficients of linear expansion.

So, the increase in surface area of 1 m² surface area of a solid for the rise of temperature 1K is called the coefficient of superficial expansion of the material of that solid. In case of expansion of a solid, normally linear expansion coefficient is generally employed. Its unit is K^-1. The coefficient of superficial expansion of copper is 33.4 x 10^-6 K^-1. We can express it in this method that it is the fractional change in length or volume per unit change in temperature. It means that if the temperature of a copper body is increased through 1K, then the increase in surface area of copper is 33.4 x 10^-6 m².