The stationary wave can be produced for both transverse and longitudinal waves. If two ends of a string are fastened straight and if, at a point, is pulled perpendicular to the length and released, then the transverse wave will be generated in the string. This wave after reflecting at the two closed ends will come back and superpose with the principal wave. After some time these two waves will also stop. This type of wave is called the stationary wave.
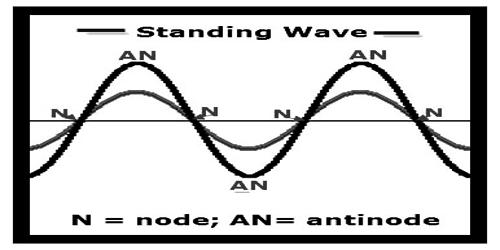
The resultant wave produced by the superposition of two progressive waves having same wavelength and amplitude, travelling in opposite direction is called the stationary wave. This wave remains confined in that particular region the wave medium and does not propagate through the medium. It can be said that remaining confined to this position, kinetic energy (elastic) is successively changed into potential energy.

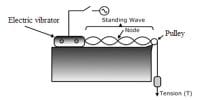
Example: When a stretched string is struck at a point, a wave is generated [Figure] and this wave propagates towards the two ends of the string. Finally, after reflection, these two waves return. Although nature of the reflected and the principal wave is same, the phase difference between them is 1800, as a result, the reflected wave and oppositely directed (new) the principle wave from the stationary wave. This wave does not go beyond the string and is produced and disappear successively in the string. If it is observed carefully, it will be seen that amplitudes at all the points of the string are not same.
Antinode: In a stationary wave amplitude of the particles at some points is zero and at some points it is maximum. The points where the amplitude is maximum is called antinodes.
Nodes: The point where the amplitude is zero is called nodes.