Equation of Motion of a Horizontal Projectile

Equation of motion of a horizontal projectile

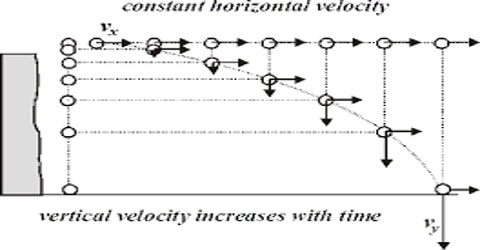
Let an object be thrown from a point O with velocity v₀ along the horizontal direction [Figure]. If we ignore air resistance and consider g constant, then along the path of motion of the thrown object v₀ will remain constant. Let after t sec the object reach at point P travelling distances x and y, respectively along horizontal and vertical directions. Now let the velocity at P be v. The horizontal and vertical components of v are respectively v_x and v_y.

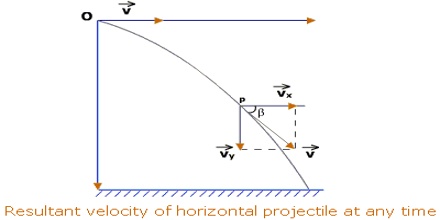
So, v_x = v₀ = v cos θ

and v_y = v₀ + gt = 0 + gt = v + sin θ

[as we know, v₀ = 0 along vertical direction]

so, v = √(v_x² + v_y²)

here angle θ in between v and horizontal direction, i.e., along X-asis,

and, tan θ = v_y/v_x

Again, x = v₀ + t … … … (1)

[a_x = 0 along horizontal direction]

so, t = x/v₀

and, y = ½ gt² …. …. …. (2)

[v₀ = 0 along vertical direction]

Now, inserting the value of t from equation (1) in equation (2) we get,

y = ½ g(x/v₀)²

so, x² = (2v₀²/g).y … … … (3)

Since, v₀ and g are constant, if we put 2v₀²/g = 4A, we get,

x² = 4A.y

This is an equation of parabola. So, trajectory of a body or a projectile ejected horizontally in resistant free-path forms a parabola.