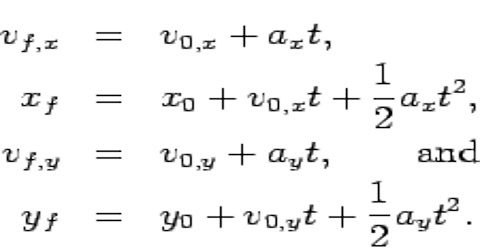Second Equation of Dimensional Motion

Second Equation of Dimensional Motion

In one dimensional motion a body moves along a straight line. So quantities associated with motion, for example displacement, velocity, acceleration etc., have only one component (moving along X-axis will have X-component and Y and Z components will be zero). In deriving equations of linear motion we will consider that the body is moving along X-axis. In that case subscripts associated with different quantifies of motion may be omitted. Normally, v_x will be represented by v and a_x by a.

Second Equation:

Equation of motion relating final velocity, acceleration and time (v = v₀ + at or v_x = v_x0 + a_xt)

Derivation: Let an object’s velocity at time, t = 0 be represented by v₀ and at a later time t, its velocity is v.

Now the change of velocity is v – v₀ during a time t – 0; its average acceleration during that time is

a = (v – v₀)/( t – 0)

Since the object is moving with uniform acceleration, so “a” is equal to the instantaneous acceleration “a”.

so, a = (v – v₀)/ t

or, (v – v₀) = at

or, v = v₀ + at … … … (1)

If initial velocity of the object is zero; i.e., the object starts from rest, then v₀ = 0. So equation (1) become

v = at

For one dimensional motion, say along X-axis, let, v₀ = v_x0; v = v_x and a = a_x; then we get from equation (1):

v_x = v_x0 + a_xt

Similarly, for motion along Y and Z-axis equation (1) reduces to,

v_y = v_y0 + a_yt

v_z = v_z0 + a_zt

Instead of uniform acceleration if the objects moves with uniform retardation, then “-a” should be inserted in the above equation.